
If that's the case I would prefer to use different symbols. Wouldn't g usually denote a coordinate transformation? The only answer I can think of is extremely ugly to me: Because we intend to evaluate that function at g(x). For example, why is the partial derivative of f with respect to the jth variable denoted by \partial f/\partial g_j all of a sudden. If you treat dr and d\theta like ordinary numbers, you will get dA=\frac, where the g_j are defined by g(x)=(g_1(x),\dots,g_n(x)).
For instance let's say you wanted to find the infinitesimal area element in polar coordinates, which isĭA=dxdy=d(rcos\theta)d(rsin\theta)=(drcos\theta-rsin\theta d\theta)(drsin\theta+rcos\theta d\theta). But you have to be more careful in multivariable calculus. The derivative of a function represents an infinitesimal change in the. Why it's true: the dy in the numerator of dy/dx is not the same dy as the one in the denominator of dx/dy.In single variable calculus things usually work out if you just assume infinitesimals work like ordinary numbers. It uses product quotient and chain rule to find derivative of any function. If dy/dx was a mere fraction, that would be shocking. That's right! In this situation ( | e1| = | e2| ): dy/dx = dx/dy, without necessarily having to be equal to 1. For the sake of visualization, let's imagine that the angle between them, theta, is equal to 60 degrees.ī) Because the vectors have the same magnitude, we can say that cos( theta ) = cos( e1, e2 ) = dy/dx = dx/dy = 0.5 ( cos(60) ) A better way to think of dy/dx is to think of it as a function, instead, where you would plug in a dx, get an intermediate dy, and then return the ratio of dy/dx.Īn example where the fraction analogy breaks down:Ī) Picture two basis vectors, e1 and e2, that have the same magnitude ( but not necessarily unit-length ), and that are NOT orthogonal ( they are oblique to one another ). The condition is that dy is the change in y ( which we call dy ) CAUSED by a change in x ( dx ). What it is: dy/dx is a fraction with a condition built in! It's VERY misleading to regard dy/dx as a mere fraction, and I believe this is one of the major pitfalls of Leibniz notation.

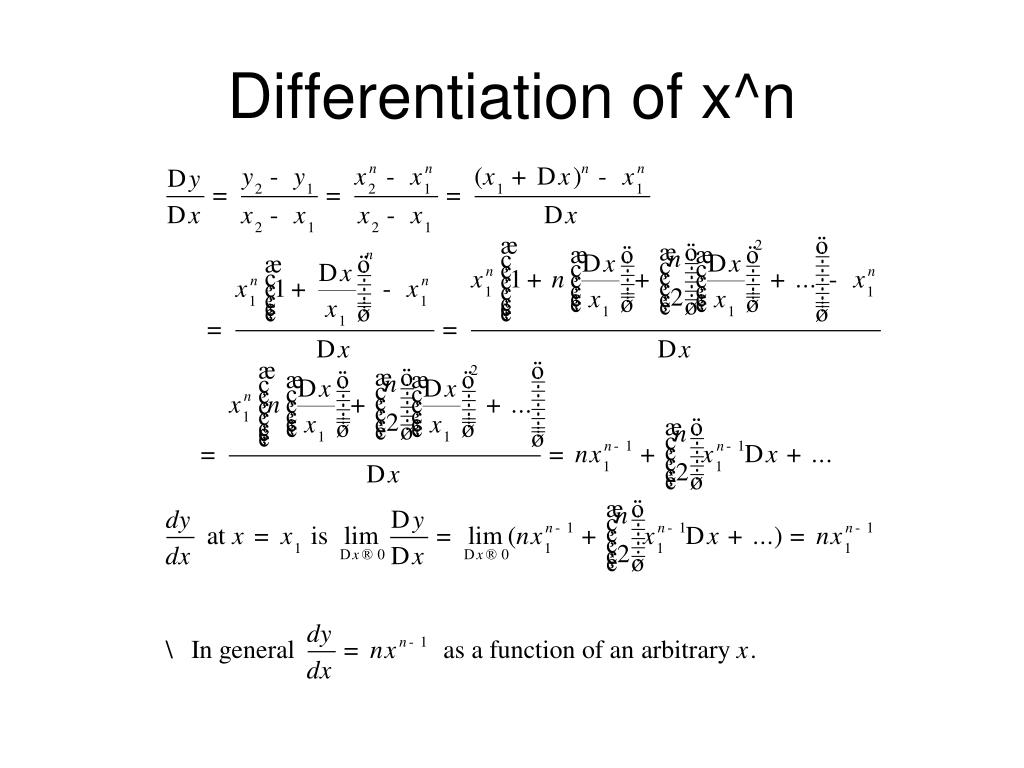
In my opinion, the only reason for why we mathematicians prefer the notation f'(x) is because we are used to the notation $g\circ f$ for the composition of two maps, which would be another important matter for discussion. (4) Analogously for the notation udv when integrating by parts. (3) Finally, this has nothing to do with infinitesimals, excepting that the derivative dy/dx is the limit of Δy/Δx when Δx->0. Moreover, it shows very well the idea that we have a linear function approximating the original f, with slope f'(x), but we are changing coordinates in order to put the origin at the point (x, y=y(x)). The formula dy=f'(x)dx is coherent with the theory of differential forms, that is, we have two 1-forms related by a function at each point. (2) It then alllows us to write dy=f'(x)dx, which is coherent with using dy/dx as if it was a fraction. Here dy/dx only means the derivative of the function y=y(x). Hence, instead of the cumsy way of differentiating y=sin (x+1) by steps one can think of y=sin z, with z=x+1 and apply dy/dx= dy/dz. So, d/dx is another notation for the derivative, and df/dx is preferable to f'(x) because it points out what variable we are using. (1) It seems to me that it would better to think of (d/dx)(y) instead of dy/dx in order to avoid misinterpretations. However, I never thought of this as a fraction. I like the notation dy/dx because many formulas and computations become more clear and easy. And, if anyone is concerned for its validity, it had a further review in Mathematics Magazine 92(5), pp.
#Infinitesimals to derive chain rule series#
You can see more details of this in "Extending the Algebraic Manipulability of Differentials", Dynamics of Continuous, Discrete and Impulsive Systems, Series A: Mathematical Analysis 26(3):217-230, 2019. Third and higher derivatives are even uglier, because you are taking the derivative of that. When written this way, the second derivative can be considered actual fractions just like the first derivative. An interesting pedagogy question came up: How misleading is it to regard $\frac$$ I am teaching Calc I, for the first time, and I haven't seriously revisited the subject in quite some time.


 0 kommentar(er)
0 kommentar(er)
